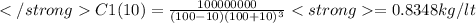Question

Initially tank I contains 100 litres of salt brine with a concentration of 1 kilogram per litre, and tank II contains 100 litres of water. Liquid is pumped from tank I into tank II at a rate of 1 litre per minute, and liquid is pumped from tank II into tank I at a rate of 2 litres per minute. The tanks are kept well stirred. Let A1 be the amount of salt in kilograms in tank I and A2 be the amount of salt in pounds in tank II.

(a) Calculate A1(t) and C1(t). For which range of values of t are the expression for A1(t) and C1(t) valid?

(b) What is the concentration in tank I after 10 minutes?

Answer 1

a)
`<strong>A1(t)=(100000000)/((100-t)(100+t)^(2) ) </strong>\\<strong>C1(t)=(A1(t))/(100+t)</strong>`

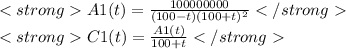
b) C1 = 0.8348 [kg/lt]

Explanation:

Explanation

First of all, the rate of change of the amount of salt in the tank I is equal to the rate of change of salt incoming less the rate change of the salt leaving, so:

`(dA1(t))/(dt)= R_(in)C_(in)-R_(out)C_(out)`

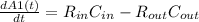
We know that the incoming rate is greater than the leaving rate, this means that the fluid in the tank I enters more than It comes out, so the total rate is :

`R_(total)=R_(in)-R_(out)=(2 lt)/(min) - (1 lt)/(min)=  (1 lt)/(min)`

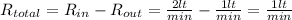
This total rate means that 1 lt of fluid enters each minute to the tank I from the tank II, with the total rate we can calculate the volume in the tank I y tank II as:

`V_(I)=100 lt + Volumen_(in)=  100 lt + ((1lt)/(min))(t) =100+t`

`V_(II)=100 lt - Volumen_(out)=  100 lt - ((1lt)/(min))(t) =100-t`

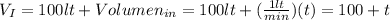
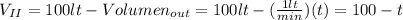
Now we have the volume of both tanks, the next step is to calculate the incoming and leaving concentration. The concentration is the ratio between the amount of salt and the volume, so:

`C(t)=C_(out) =(A1(t))/(V_(I) )=(A1(t))/(100+t )`

Since fluid is pumped from tank I into tank II, the concentration of the tank II is a function of the amount of salt of the tank I that enters into the tank II, thus:

`C_(in) =((A1(t)/V_(I))(t))/(V_(II) )=(A1(t))/(V_(I) V_(II))(t)`

`C_(in) =(A1(t))/((100+t)(100-t))(t)=(A1(t))/((10000-t^(2) ))(t)`

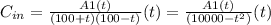
If we substitute the concentrations and the rates into the differential equation we can get:

`(dA1(t))/(dt)= R_(in)C_(in)-R_(out)C_(out)\\(dA1(t))/(dt)= (2)(((t)A1(t))/(10000-t^(2) ))-(1)(((A1(t))/(100+t ))`

`(dA1(t))/(dt)= A1(t)((2t)/(10000-t^(2) )-(1)/(100+t ))`

`(dA1(t))/(dt)- ((2t)/(10000-t^(2) )-(1)/(100+t ))A1(t)=0`

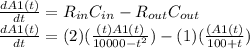
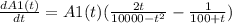
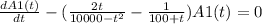
The obtained equation is a homogeneous differential equation of first order and the solution is:

a)
`A1(t)= (100000000)/((100-t)(100+t)^(2) )`

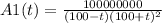
and the concentration is:

`C1(t)= (100000000)/((100-t)(100+t)^(3))`

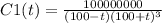
This equations A1(t) and C1(t) are only valid to 0<=t<100 because to t >=100 minutes the tank II will be empty and mathematically A1(t>=100) tends to the infinite.

b) To calculate the concentration in the tank I after 10 minutes we have to substitute t=10 in C1(t), thus:

`</strong>C1(10)= (100000000)/((100-10)(100+10)^(3))<strong>=0.8348 kg/lt`