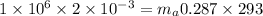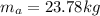Answer:
The air mass in the tank is 23.78 kg
Solution:
As per the question:
Volume of the tank,
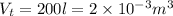
Pressure, P = 1 MPa =
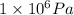
Temperature, T =
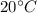 = 273 + 20 = 293 K
= 273 + 20 = 293 K
Pressure, P' = 0.5 MPa =

Now,
To calculate the air mass,
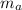 we use:
we use:
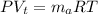
where
R = Rydberg constant = 0.287 J/kg.K