Question

Let V be the set of pairs (x; y) of real numbers and let the eld F be the

real number set. Dene the addition and scalar multiplication as follows:

(x1; y1) + (x2; y2) = (x1 + x2; 0)

c(x; y) = (cx; 0):

Is V , with these operations, a vector space? Explain.

Answer 1

To prove that V is a vector space we must prove that the sum define on it satisfy conmutativiy, asociativity and existence of the neutral element and inverses. Also, the scalar multiplication define on V must satisfy distributivity propertie with respect to the sum and viceversa, and an asosiativity too in the sense that
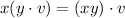`x(y\cdot v)= (xy)\cdot v` for
`x,y\in \mathbb{R}` and
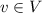`v\in V`. One can prove with this that the neutral element for the sum is unique. But with your operations you have two neutral elements for
`(1;2)`

`(1;2)+(-1;3)=(0;0)`

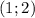
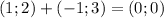
and

`(1;5)+(-1;11)=(0;0)`

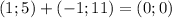
So, you dont have a vector space.