Answer:
Explanation:
1. Number of boys in the group = 25
Number of girls in the group = 18
Total children = 25 + 18 = 43
Number of ways to arrange the children in a way = 43!
2. If we consider all the boys as an individual then number of ways children can be arranged = 19!
Number of ways boys can sit next to each other = 25!
So the number of ways can be arranged = 19!×25!
3. Number of ways boys can sit next to each other = 25!
Number of ways girls can sit next to each other = 19!
Then number of ways to arrange the children in a row with all boys next to each other and all the girls next to each other will be = 2 × 18! × 25!
4. 1. To choose a chess team if anyone can be chosen
=
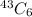
= 6096454
4. 2. Exactly 2 girls must be chosen then number of ways
=
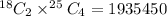
4. 3. At least two boys must be chosen
=

= 5863690