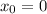Answer:
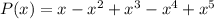
Explanation:
Let us first remember how a Taylor polynomial looks like:
Given a differentiable function
 then we can find its Taylor series to the
then we can find its Taylor series to the
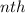 degree as follows:
degree as follows:

Where
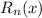 represents the Remainder and
represents the Remainder and
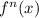 is the
is the
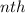 derivative of
derivative of
 .
.
So let us find those derivatives.
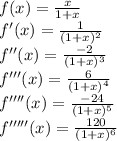
The only trick for this derivatives is for the very first one:
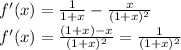
Then it's only matter of replacing on the Taylor Series and replacing