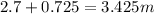Answer:
The distance of spot of light from his feet equals 3.425 meters.
Step-by-step explanation:
The situation is represented in the attached figure below
The angle of incidence is computed as
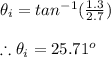
Now by Snell's law we have
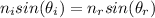
where
 are the refractive indices of the incident and the refracting medium respectively
are the refractive indices of the incident and the refracting medium respectively
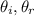 are the angle of incidence and the angle of refraction respectively
are the angle of incidence and the angle of refraction respectively
Thus using the Snell's relation we have

from the attached figure we can see
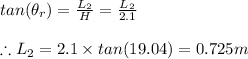
Thus distance of spot on the pool bed from his feet equals