Answer:
I think that what you are trying to show is: If
 is irrational and
is irrational and
 is rational, then
is rational, then
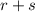 is rational. If so, a proof can be as follows:
is rational. If so, a proof can be as follows:
Explanation:
Suppose that
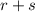 is a rational number. Then
is a rational number. Then
 and
and
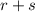 can be written as follows
can be written as follows
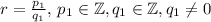
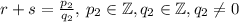
Hence we have that
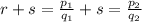
Then
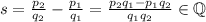
This is a contradiction because we assumed that
 is an irrational number.
is an irrational number.
Then
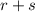 must be an irrational number.
must be an irrational number.