Answer:
after 38.8 years it will double
correct option is D 38.8 years
Step-by-step explanation:
given data
population grows rate = 1.8%
to find out
how many years will it take to double
solution
we consider here initial population is x
so after 1 year population will be = (100% + 1.8% ) x = 1.018 x
and after n year population will be =
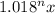
so it will double
2x =
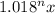
take log both side
log 2 = n log (1.018)
n =
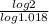
n = 38.853
so after 38.8 years it will double
correct option is D 38.8 years