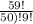Answer:
number of way is
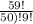
Explanation:
given data:
total number of problems 100
total points for each problem 5
let ten problems are
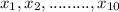
according to the given information

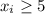
where i =1 + 10
so, number of way integer point can assign are
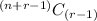
where
r = 10

so, we have
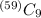
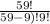
number of way is