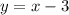Answer:
The equation of the line is:
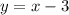
Explanation:
The general equation of a straight line is given by:
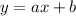
Being given two points, we can replace x and y, solve the system and find the values for a and b.
Solution:
The line goes through the point
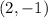 . It means that when
. It means that when
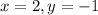 . Replacing in the equation:
. Replacing in the equation:
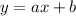
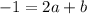
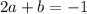
The line also goes through the point
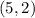 . It means that when
. It means that when
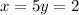 . Replacing in the equation:
. Replacing in the equation:
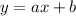
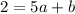
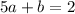
Now we have to solve the following system of equations:
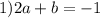
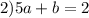
From 1), we have:

Replacing in 2)


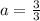
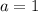
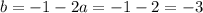
The equation of the line is: