Question

Xy′ = √(1 − y2 ), y(1) = 0

Answer 1

The particular solution is
`y=\sin (\ln|x|)` .

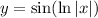Explanation:

The given differential equation is

`xy'=\sqrt {1-y^2}`

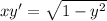
It can be written as

`x(dy)/(dx)=\sqrt {1-y^2}`

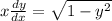
Use variable separable method to solve the above equation.

`\frac{dy}{\sqrt {1-y^2}}=(1)/(x)dx`

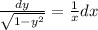
Integrate both sides.

`\int \frac{dy}{\sqrt {1-y^2}}=\int (1)/(x)dx`

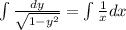
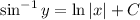`\sin^(-1) y=\ln|x|+C` .... (1)

It is given that y(1)=0. It means y=0 at x=1.

`\sin (0)=\ln|1|+C`

`0=0+C`

`0=C`

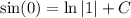
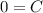
The value of constant is 0.

Substitute C=0 in equation (1) to find The required equation.

`\sin^(-1) y=\ln|x|+0`

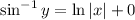
Taking sin both sides.

`y=\sin (\ln|x|)`

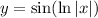
Therefore the particular solution is
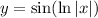`y=\sin (\ln|x|)` .