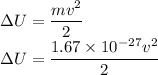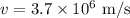Answer:
A)
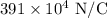
B
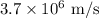
Step-by-step explanation:
Given:
- Diameter of each disc , d=2.3 cm
- Magnitude of total Charge on each disc Q=16 nC
A)Let
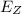 Electric Field due to a disc of Radius R containing charge Q at a distance Z from its centre in direction perpendicular to the plane of the disc given by
Electric Field due to a disc of Radius R containing charge Q at a distance Z from its centre in direction perpendicular to the plane of the disc given by
since the disks are identical and charges on them are same and and distance of point from their centres are also same so the Electric field at P will be twice the Electric Field due to one.
 )\\\\
)\\\\
The Direction of Electric Field will be from disk containing positive charge towards negative charge.
B))Let
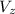 Electric potential due to a disc of Radius R containing charge Q at a distance Z from its centre in direction perpendicular to the plane of the disc given by
Electric potential due to a disc of Radius R containing charge Q at a distance Z from its centre in direction perpendicular to the plane of the disc given by
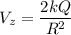
At the centre of both the disks z=0
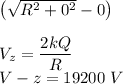
At z=0.0029 m

since the disks are identical and charges on them are same and and distance of point from their centres are also same so the Electric potential at P will be twice the Electric Field due to one.
So the change in Electric potential is
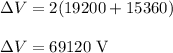
Change in potential Energy is
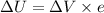
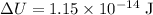
The change in potential Energy will be equal to the the change in kinetic Energy of the proton