Question

Suppose you take a 50gram ice cube from the freezer at an initial temperature of -20°C. How much energy would it take to completely vaporize the ice cube? (Hint: think of this process as four separate steps and calculate the energy needed for each one.)

Answer 1

It would take 200 kJ of energy to completely vaporize the ice cube.

Step-by-step explanation:

The process of completely vaporizing an ice cube can be broken down into four separate steps, each requiring a certain amount of energy:

1. Heating the ice cube from -20°C to 0°C: This step requires 4.1 kJ of energy.
2. Melting the ice at 0°C: This step requires 133.6 kJ of energy.
3. Heating the resulting water from 0°C to 37°C: This step requires 61.9 kJ of energy.
4. Vaporizing the water at 37°C: This step requires an additional 0 kJ of energy, since the water is already at its boiling point.

In total, it would take 200 kJ of energy to completely vaporize the ice cube.

Answer 2

The amount of energy required is
`152.68* 10^(3)Joules`

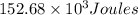
Step-by-step explanation:

The energy required to convert the ice to steam is the sum of:

1) Energy required to raise the temperature of the ice from -20 to 0 degree Celsius.

2) Latent heat required to convert the ice into water.

3) Energy required to raise the temperature of water from 0 degrees to 100 degrees

4) Latent heat required to convert the water at 100 degrees to steam.

The amount of energy required in each process is as under

1)
`Q_1=mass* S.heat_(ice)* \Delta T\\\\Q_1=50* 2.05* 20=2050Joules`

where

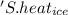`'S.heat_(ice)`' is specific heat of ice =
`2.05J/^(o)C\cdot gm`

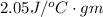
2) Amount of heat required in phase 2 equals

`Q_2=L.heat* mass\\\\\therefore Q_(2)=334* 50=16700Joules`

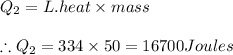
3) The amount of heat required to raise the temperature of water from 0 to 100 degrees centigrade equals

`Q_3=mass* S.heat* \Delta T\\\\Q_1=50* 4.186* 100=20930Joules`

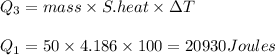
where

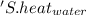`'S.heat_(water)`' is specific heat of water=
`4.186J/^(o)C\cdot gm`

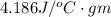
4) Amount of heat required in phase 4 equals

`Q_4=L.heat* mass\\\\\therefore Q_(4)=2260* 50=113000Joules\\\\`
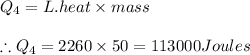
`\\\\\\\\`Thus the total heat required equals
`Q=Q_(1)+Q_(2)+Q_(3)+Q_(4)\\\\Q=152.68* 10^(3)Joules`