Question

A particle with a charge of -60.0 nC is placed at the center of a nonconducting spherical shell of inner radius 20.0 cm and outer radius 33.0 cm. The spherical shell carries charge with a uniform density of-1.30 μC/m^3. A proton moves in a circular orbit just outside the shcal shell. Calculate the speed of the proton.

Answer 1

Step-by-step explanation:

Total volume of the shell on which charge resides

= 4/3 π ( R₁³ - R₂³ )

= 4/3 X 3.14 ( 33³ - 20³) X 10⁻⁶ m³

= 117 x 10⁻³ m³

Charge inside the shell

-117 x 10⁻³ x 1.3 x 10⁻⁶

= -152.1 x 10⁻⁹ C

Charge at the center

= - 60 x 10⁻⁹ C

Total charge inside the shell

= - (152 .1 + 60 ) x 10⁻⁹ C

212.1 X 10⁻⁹C

Force between - ve charge and proton

F = k qQ / R²

k = 9 x 10⁹ .

q = 1.6 x 10⁻¹⁹ ( charge on proton )

Q = 212.1 X 10⁻⁹ ( charge on shell )

R = 33 X 10⁻² m ( outer radius )

F =
`(9*10^9*1.6*10^(-19)*212.1* 10^(-9))/((33*10^(-2))^2)`

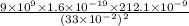
F = 2.8 X 10⁻¹⁵ N

This force provides centripetal force for rotating proton

mv² / R = 2.8 X 10⁻¹⁵

V² = R X 2.8 X 10⁻¹⁵ / m

= 33 x 10⁻² x 2.8 x 10⁻¹⁵ /( 1.67 x 10⁻²⁷ )

[ mass of proton = 1.67 x 10⁻²⁷ kg)

= 55.33 x 10¹⁰

V = 7.44 X 10⁵ m/s