Question

How is the principle of superposition used to determine the stress state for a combined loading?

Answer 1

Answer with Explanation:

For a linearly responsive system principle of superposition states that

"The cumulative response of the given system to forces of different magnitudes is the sum of the individual responses of the system to the individual forces"

In a less formal manner principle of superposition states that the effect of various forces acting together on any body is the sum of all the effects on the body produced when each force acts individually.

Now we know that

`Stress=(Force)/(Area)`

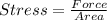
Since stress at any point is in linear relation with the force hence we can conclude that the state of stress at any point due to different forces acting together is the sum of the individual stresses due to individual forces alone.

Mathematically

Let the stress due to a force
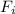`F_i` be
`\sigma _i`

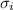
and the stress due to combined forces be
`\sigma _f`

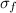
thus according to principle of superposition we have

`\sigma_f=\sum_(i=1)^(n)(\sigma_i)`