Question

Triangle ABC has a perimeter of 12 units. The vertices of the triangle are A(x,2), B(2,-2), and C(-1,2). Find the value of x.

Answer 1

The value of x is 2

Explanation:

* Lets explain how to find a distance between 2 points

- If the endpoints of a segment are
`(x_(1),y_(1))` and

`(x_(2),y_(2))` is
`d=\sqrt{(x_(2)-x_(1))^(2)+(y_(2)-y_(1))^(2)}`

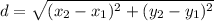
- Triangle ABC has a perimeter of 12 units

∵ The perimeter of any triangle is the sum of lengths of its sides

∴ P Δ ABC = AB + BC + AC

* Lets find the length of the three sides

∵ A = (x , 2) , B = (2 , -2) , C = (-1 , 2)

∵
`AB=\sqrt{(2-x)^(2)+(-2-2)^(2)}`

∴
`AB=\sqrt{(2-x)^(2)+(-4)^(2)}`

∴
`AB=\sqrt{(2-x)^(2)+16}`

∵
`BC=\sqrt{(-1-2)^(2)+(2--2)^(2)}`

∴
`BC=\sqrt{(-3)^(2)+(4)^(2)}`

∴
`BC=√(9+16)`

∴
`BC=√(25)`

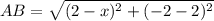
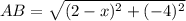
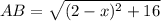
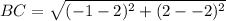
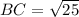
∴ BC = 5

∵
`CA=\sqrt{(x--1)^(2)+(2-2)^(2)}`

∴
`CA=\sqrt{(x+1)^(2)+(0)^(2)}`

∴
`CA=\sqrt{(x+1)^(2)}`

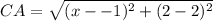
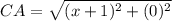
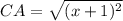
- The √ is canceled by power 2

∴ CA = (x + 1)

∵ AB + BC + CA = 12

∴
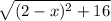`\sqrt{(2-x)^(2)+16}` + 5 + (x + 1) = 12

- Add 5 and 1

∴
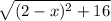`\sqrt{(2-x)^(2)+16}` + 6 + x = 12

- subtract 6 and x from both sides

∴
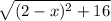`\sqrt{(2-x)^(2)+16}` = (6 - x)

- To cancel (√ ) square the two sides

∴ (2 - x)² + 16 = (6 - x)²

- Simplify the two sides

∴ [(2)(2) + (2)(2)(-x) + (-x)(-x)] + 16 = (6)(6) + (2)(6)(-x) + (-x)(-x)

∴ 4 - 4x + x² + 16 = 36 - 12x + x²

- Subtract x² from both sides

∴ 20 - 4x = 36 - 12x

- Add 12x to both sides and subtract 20 from both sides

∴ 12x - 4x = 36 - 20

∴ 8x = 16

- Divide both sides by 8

∴ x = 2

* The value of x is 2