Question

(a) With what speed must a ball be thrown vertically from ground level to rise to a maximum height of 52 m? (b) How long will it be in the air?

Answer 1

Step-by-step explanation:

Maximum height reached by the ball, s = 52 m

Let u is the initial speed of the ball and v is the final speed of the ball, v = 0 because at maximum height the final speed goes to 0. We need to find u.

(a) The third equation of motion as :

`v^2-u^2=2as`

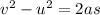
Here, a = -g

`0-u^2=-2gs`

`u^2=2* 9.8* 52`

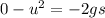
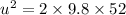
u = 31.92 m/s

(b) Let t is the time when the ball is in air. It is given by :

`v=u+at`

`u=gt`

`t=(31.92\ m/s)/(9.8\ ms/^2)`

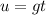
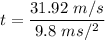
t = 3.25 seconds

Hence, this is the required solution.