Answer: About 16 years
Explanation:
The formula to find the compound amount if compounded continuously is given by :-
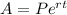 , where P is Principal amount, r is the rate of interest ( in decimal) and t is time ( in years).
, where P is Principal amount, r is the rate of interest ( in decimal) and t is time ( in years).
Given : P= $1000 ; r= 4.6%=0.046
let t be the time it will take to double the amount, the we have
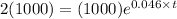
Dividing 1000 both sides, we get
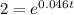
Taking natural log on each side, we get
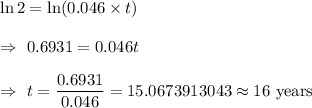
Hence, it will take about 16 years to double the amount.