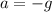Question

1. A man stands on top of a 25 m tall cliff. The man throws a rock upwards at a 25-degree angle above the horizontal with an initial velocity of 7.2 m/s. What will be the vertical position of the rock at t = 2 seconds? What will be the horizontal position of the rock at that time?

2. A cannon fires a cannonball forward at a velocity of 48.1 m/s horizontally. If the cannon is on a mounted wagon 1.5 m tall, how far would the cannonball travel before it lands on the ground?
3. Look at the following picture. What would be the resultant vector of A+B?

4. An airplane undergoes the following displacements: First, it flies 72 km in a direction 30° east of north. Next, it flies 48 km due south. Finally, it flies 100 km 30° north of west. Using analytical methods, determine how far the airplane ends up from its starting point.
5.The following picture shows a golf ball being hit and given an initial velocity of v0. The ball is hit at an unknown angle above the ground. What are TWO values that are known, even in the absence of all other numbers?

Answer 1

These are the answers for 1, 2 and 3

Step-by-step explanation:

Sorry I couldn't help you with 4 and 5

Answer 2

1.

Answer:

y = 11.48 m

x = 13.0 m

Step-by-step explanation:

Components of initial velocity is given as

`v_x = 7.2 cos25 = 6.52 m/s`

`v_y = 7.2 sin25 = 3.04 m/s`

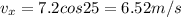
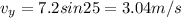
Now after t = 2 s the vertical position is given as

`y = y_0 + v_y t + (1)/(2)a_y t^2`

`y = 25 + 3.04(2) - (1)/(2)(9.8)(2^2)`

`y = 11.48 m`

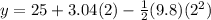
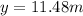
Now horizontal position is given as

`x = v_x t`

`x = 6.52 * 2`

`x = 13.04 m`

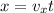
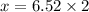
2.

Answer:

d = 26.6 m

Step-by-step explanation:

Initial position on y axis is given as

`y = 1.5 m`

velocity of ball in y direction

`v_y = 0`

now we have

`\Delta y = v_y t + (1)/(2)gt^2`

`1.5 = (1)/(2)(9.8) t^2`

`t = 0.55 s`

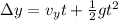
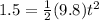
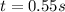
now the distance moved by the ball in horizontal direction is given as

`d = v_x t`

`d = 48.1 * 0.55`

`d = 26.6 m`

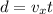
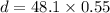
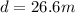
3

Answer:

`A + B = 12.42\hat i + 0.35 \hat j`

Step-by-step explanation:

Here we can see the two vectors inclined at different angles

so two components of vector A is given as

`A = 11.3 cos21\hat i - 11.3 sin21\hat j`

`A = 10.55 \hat i - 4.05 \hat j`

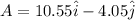
Similarly for other vector B we have

`B = 4.78cos67 \hat i + 4.78 sin67\hat j`

`B = 1.87\hat i + 4.4 \hat j`

now we need to find A + B

so we have

`A + B = (10.55\hat i - 4.05\hat j) + (1.87\hat i + 4.4 \hat j)`

`A + B = 12.42\hat i + 0.35 \hat j`

4.

Answer:

d = 81.86 m

Step-by-step explanation:

Displacement of airplane is given as

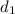`d_1` = 72 km in direction 30 degree East of North

`d_1 = 72sin30\hat i + 72cos30\hat j`

`d_1 = 36\hat i + 62.35\hat j`

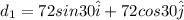
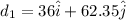
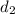`d_2` = 48 km South

`d_2 = -48\hat j`

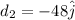
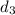`d_3` = 100 km in direction 30 degree North of West

`d_3 = -100 cos30\hat i + 100 sin30\hat j`

`d_3 = -86.6\hat i + 50\hat j`

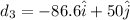
so net displacement is given as

`d = d_1 + d_2 + d_3`

`d = 36\hat i + 62.35\hat j - 48\hat j - 86.6\hat i + 50 \hat j`

`d = -50.6\hat i + 64.35\hat j`

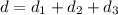
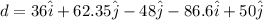
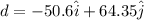
now magnitude of displacement is given as

`d = √(50.6^2 + 64.35^2)`

`d = 81.86 m`

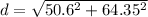
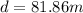
5.

Answer:

1) final speed at which it will hit the ground again

2) acceleration during the motion of the ball

Step-by-step explanation:

As we know that the speed at which the ball is thrown is always same to the speed by which it will hit back on the ground

so we know that final speed will be same as initial speed

Also we know that during the motion the acceleration of ball is due to gravity so it will be

`a = - g`