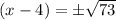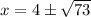Question

Use completeling the square to solve for x in the equation (x-12)(x+4)=9

Answer 1

x=
`4\pm √(73)`

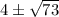
Explanation:

First step. Solve the binomial product from the left side of the equation:

`(x-12)(x+4)=9`

`x^2+4x-12x-48=9`

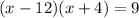
Second step. Simplify and move independent terms to the right side of the equation:

`x^2-8x=57`

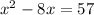
Third step. Find a number that multiplied by two gives -8, then square this number and sum it on both sides of the equation:

`x^2-8x+16=57+16`

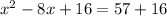
Fourth step. Write the left side of the equation as a squared binomial:

`(x-4)^2=73`

Fifth step. First, take the square root and then add 4 to both sides of the equation to solve for x:

`(x-4)=\pm √(73)`

`x= 4\pm √(73)`