Question

Find P-1, where P = [adg beh cfi] is orthogonal.

Answer 1

Answer: The required inverse of the given matrix is

`P^(-1)=\left[\begin{array}{ccc}a&b&c\\d&e&f\\g&h&i\end{array}\right].`

Step-by-step explanation: We are given to find the inverse of the following orthogonal matrix :

`P=\left[\begin{array}{ccc}a&d&g\\b&e&h\\c&f&i\end{array}\right] .`

We know that

if M is an orthogonal matrix, then the inverse matrix of M is the transpose of M.

That is,
`M^(-1)=M^T.`

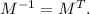
The transpose of the given matrix P is given by

`P^T=\left[\begin{array}{ccc}a&b&c\\d&e&f\\g&h&i\end{array}\right].`

Therefore, according to the definition of an orthogonal matrix, the inverse of matrix P is given by

`P^(-1)=P^T=\left[\begin{array}{ccc}a&b&c\\d&e&f\\g&h&i\end{array}\right].`

Thus, the required inverse of the given matrix is

`P^(-1)=\left[\begin{array}{ccc}a&b&c\\d&e&f\\g&h&i\end{array}\right].`