Question

Find all solutions of the equation algebraically. Check your solutions. (Enter your answers as a comma-separated list x^4-7x^2-144=0

Answer 1

The solutions are:
`x=4,\:x=-4,\:x=3i,\:x=-3i`

Explanation:

Consider the provided equation.

`x^4-7x^2-144=0`

Substitute
`u=x^2\mathrm{\:and\:}u^2=x^4`

`u^2-7u-144=0`

`u^2-16u+9u-144=0`

`(u-16)(u+9)=0`

`u=16,\:u=-9`

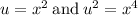
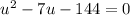
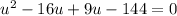
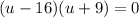
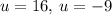
Substitute back
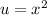`\:u=x^2` and solve for x.

`x^2=16\\x=√(16)\\ \quad x=4,\:x=-4`

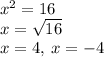
Or

`x^2=-9\\x=√(-9)\\ \quad x=3i,\:x=-3i`

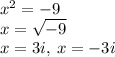
Hence, the solutions are:
`x=4,\:x=-4,\:x=3i,\:x=-3i`

Check:

Substitute x=4 in provided equation.

`4^4-7(4)^2-144=0`

`256-112-144=0`

`0=0`

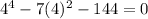
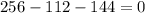
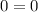
Which is true.

Substitute x=-4 in provided equation.

`(-4)^4-7(-4)^2-144=0`

`256-112-144=0`

`0=0`

Which is true.

Substitute x=3i in provided equation.

`(3i)^4-7(3i)^2-144=0`

`81+63-144=0`

`0=0`

Which is true.

Substitute x=-3i in provided equation.

`(-3i)^4-7(-3i)^2-144=0`

`81+63-144=0`

`0=0`

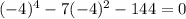
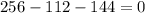
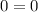
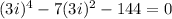
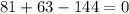
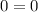
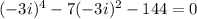
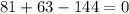
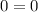
Which is true.