Question

Two coins are tossed simultaneously,Find the probability of getting.1.atleast one head.2.at most one tail​

Answer 1

If two coins are tossed simultaneously, you will have one of the possible results

`HH,\ HT,\ TH,\ TT`

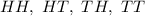
with probability 1/4 each.

So, you'll have at least one head if you have HH, HT or TH, i.e. with probability 3/4.

Similarly, you have at most one tail if you get HH, HT or TH, so the probability will be the same.

In fact, both claims (at least one head and at most one tail) are verified by the same set of outputs HH, TH, HT, since they're not satisfied only by the output HH.

So, if those claims are not satisfied with probability 1/4, they're satisfied with probability 3/4.