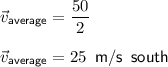Question

You travel 300 meters North, then head back 350 meters South in 2 minutes. What is the average velocity?

Answer 1

25 m/s to south

Step-by-step explanation:

Average velocity is total displacement over total time. Mathematically, it can be expressed as:

`\displaystyle{\vec{v}_{\sf{average}} = \frac{\vec{s}_{\sf{total}}}{t_{\sf{total}}}}`

Where
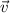`\displaystyle \vec v` is velocity,
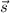`\displaystyle \vec s` is displacement and
`\displaystyle t` is time. Displacement is change in position.

The position changes from 0 meter to 300 meters north. Then it changes from 300 meters north to 350 meters south. Therefore:

`\displaystyle{\vec{s}_1 = 300 \ \, \sf{meters} \ \, \sf{north}}\\\\\displaystyle{\vec{s}_2 = 350 \ \, \sf{meters} \ \, \sf{south}}`

The direction is opposite to each others for 300 meters north and 350 meters south.

Since you have more displacement to south more than you have to north. The direction will be in south and you'll have total displacement of 350 - 300 = 50 meters to south.

Therefore:

`\displaystyle{\vec{s}_{\sf{total}} = 50 \ \, \sf{meters} \ \, \sf{south}}`

Within 2 minutes, you are able to travel displacement of 50 meters to south. Therefore, substitute in the formula:

`\displaystyle{\vec{v}_{\sf{average}} = (50)/(2)}\\\\\displaystyle{\vec{v}_{\sf{average}} = 25 \ \, \sf{m/s} \ \, \sf{south}}`