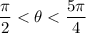Question

Sinθ> cosθ<0 where does angle θ lie

Answer 1

`\theta\in\left(\pi+2k\pi;\ (3\pi)/(4)+2k\pi\right)`

Explanation:

I think correct inequality is

`\cos\theta<\sin\theta<0`

The easiest way is to draw graphs of sine and cosine functions in one coordinate system and read a set of solutions from the graph (look at the picture).

We need to solve the equation

`\sin\theta=\cos\theta`

to get the intersection points of the graphs.

`\sin\theta=\cos\theta\qquad\text{divide both sides by}\ \cos\theta\\eq0\\\\(\sin\theta)/(\cos\theta)=1\to\tan\theta=1\to\theta=(\pi)/(4)+k\pi,\ k\in\mathbb{Z}`

We look at where the sine function graph is above the cosine function graph.

Answer 2

Since you want a negative cosine, your angle will surely lie in the II or III quadrant.

In particular, in the II quadrant, the cosine is negative and the sine is positive, so you surely have
`\sin(\theta)>\cos(\theta)`

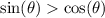
As for the third quadrant, we start with

`\sin(\pi)=0,\quad\cos(\pi)=-1`

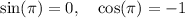
(which implies that the sine is greater than the cosine) and we end with

`\sin\left((3\pi)/(2)\right)=-1,\quad\cos\left((3\pi)/(2)\right)=0`

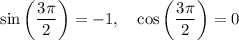
which implies that the sine is less than the cosine.

Halfway through, we have

`\sin\left((5\pi)/(4)\right)=\cos\left((5\pi)/(4)\right)=(√(2))/(2)`

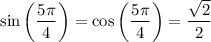
So, only the first half of the III quadrant is fine, because sine and cosine are both negative, but the sine is "less negative" than the cosine, so we have, as requested,

`\begin{cases}\sin(\theta)>\cos(\theta)\\\cos(\theta)<0\end{cases}`

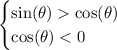
So, the final answer is that the angle lies in the interval

`(\pi)/(2)<\theta<(5\pi)/(4)`