Question

At Lamppost Pizza there are four pizza toppings: pepperoni, sausage, mushrooms, and anchovies. When you order a pizza you can have as few or as many toppings you want from the above list. You can also choose to have none of the above. How many different kinds of pizza could you order?

Please help immediately!!! :(

Answer 1

You could order 16 different kinds of pizza.

Explanation:

You have those following toppings:

-Pepperoni

-Sausage

-Mushrooms

-Anchovies

The order is not important. For example, if you choose Sausage and Mushrooms toppings, it is the same as Mushrooms and Sausage. So we have a combination problem.

Combination formula:

A formula for the number of possible combinations of r objects from a set of n objects is:

`C_((n,r)) = (n!)/(r!(n-r!)`

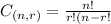
How many different kinds of pizza could you order?

The total T is given by

`T = T_(0) + T_(1) + T_(2) + T_(3) + T_(4)`

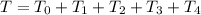
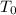`T_(0)` is the number of pizzas in which there are no toppings. So
`T_(0) = 1`

`T_(1)` is the number of pizzas in which there are one topping
`T_(1)` is a combination of 1 topping from a set of 4 toppings. So:

`T_(1) = (4!)/(1!(4-1)!) = 4`

`T_(2)` is the number of pizzas in which there are two toppings
`T_(2)` is a combination of 2 toppings from a set of 4 toppings. So:

`T_(2) = (4!)/(2!(4-2)!) = 6`

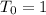
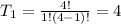
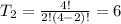
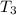`T_(3)` is the number of pizzas in which there are three toppings
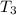`T_(3)` is a combination of 3 toppings from a set of 4 toppings. So:

`T_(3) = (4!)/(3!(4-3)!) = 4`

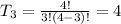
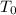`T_(0)` is the number of pizzas in which there are four toppings. So
`T_(4) = 1`

Replacing it in T

`T = T_(0) + T_(1) + T_(2) + T_(3) + T_(4) = 1 + 4 + 6 + 4 + 1 = 16`

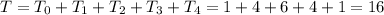
You could order 16 different kinds of pizza.