Question

The density of mercury is 13.5939 g/cm3. Calculate the mass in kilograms of a drum full of mercury with the following dimensions: height = 1.100 meters, diameter = 0.492 meters. Assume pi has a value of 3.14.

Answer 1

Mass, m = 0.00284 kg

Step-by-step explanation:

Given that,

Density of mercury,
`d=13.5939\ g/cm^3`

Height of mercury column, h = 1.1 m

Diameter of mercury, d = 0.492 meters

Radius of mercury column, r = 0.246 m

We need to find the mass of a drum. The density is given by :

`d=(m)/(V)`

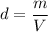
V is the volume of mercury column

`d=(m)/(\pi r^2h)`

`m=d* \pi r^2h`

`m=13.5939* 3.14* (0.246)^2* 1.1`

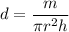
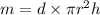
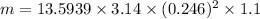
m = 2.84 grams

or

m = 0.00284 kg

So, the mass of a drum full of mercury is 0.00284 kg. Hence, this is the required solution.