Answer:

Explanation:
Given : An opaque bag contains 5 green marbles, 3 blue marbles and 2 red marbles.
Total marbles = 5+3+2=10
Now, if it given that the a red marble is already drawn, then the total marbles left in the bag = 10-1=9
But the number of blue marbles remains the same.
Now, the probability of drawing a blue marble given the first marble was red :-
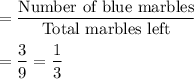
Hence, the required probability =
