Question

Bond Yields and Rates of Return A 30-year, 10% semiannual coupon bond with a par value of $1,000 may be called in 4 years at a call price of $1,100. The bond sells for $1,050. (Assume that the bond has just been issued.) What is the bond's yield to maturity? Do not round intermediate calculations. Round your answer to two decimal places.

Answer 1

The bond's yield to maturity is 9.45% using Excel to get exact values, and 9.59% using approximate method.

Step-by-step explanation:

We can calculate is using 2 ways, using Excel to get the exact percentage or with approximate methods, calculating the semi-annual Yield to Maturity using the following formula

`YTM_(sm) =\cfrac{PMT+\cfrac{FV-PV}n}{\cfrac{FV+PV}2}`

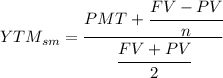
And from there we can calculate the Yield to Maturity just by multiplying the semi-annual one by 2.

Identifying the given information.

We have a period of 30 years, so for the semiannual bond we have
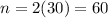`n=2(30) = 60` periods.

The face value, FV, is $1000, the coupon rate is 0.10, thus we can use them to find the interest per period PMT.

`PMT=0.10 * \cfrac{1000}{2}\\PMT=\$ 50`

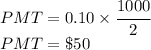
The current price of the bond, PV is $1050.

Replacing the values on the semiannual Yield to Maturity

`YTM_(sm) =\cfrac{PMT+\cfrac{FV-PV}n}{\cfrac{FV+PV}2}`

`YTM_(sm)=\cfrac{50+\cfrac{1000-1050}{60}}{\cfrac{1000+1050}{2}}`

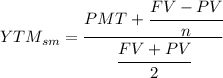
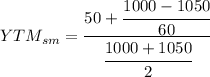
Simplifying we get

`YTM_(sm)=4.797\%\\`

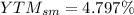
Finding the Yield to Maturity.

We can just multiply by 2 to get the Yield to Maturity from our previous result and rounding it to 2 decimals we get

`YTM = 2 YTM_(sm)\\YTM=9.59\%`

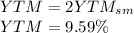
Alternatively we can use Excel and write:

RATE(n, PMT, PV, FV)*2

That is

RATE(60,50,1050,1000)*2

And we will get the exact Yield to maturity 9.49%