Question

Find the linear trend forecast for period 8 given the following data:  Period            Sales 1                     19 2                     18 3                     15 4                     20 5                     18 6                     22  7                     20

Answer 1

The equation of trend line is
`y = 0.500000x + 16.857143`.

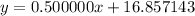The linear trend forecast for period 8 is about 20.86.

Explanation:

The given data table is

Period Sales

1 19

2 18

3 15

4 20

5 18

6 22

7 20

We need to find the linear trend forecast for period 8.

The general form of linear regression is

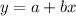`y=a+bx` .... (1)

where, a is y-intercept and b is slope.

`b=\frac{\sum_(i=1)^nx_iy_i-n\overline{x}\overline{y}}{\sum_(i=1)^nx_i^2-n\overline{x}^2}`

`a=\overline{y}-b\overline{x}`

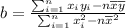
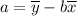
Using the graphing calculator we get

`a=16.857143`

`b=0.500000`

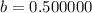
Substitute these values in equation (1).

`y = 0.500000x + 16.857143`

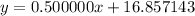
The equation of trend line is
`y = 0.500000x + 16.857143`.

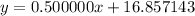Substitute x=8 to find the linear trend forecast for period 8.

`y = 0.500000(8) + 16.857143`

`y =20.857143`

`y \approx 20.86`

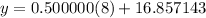
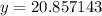
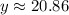
Therefore the linear trend forecast for period 8 is about 20.86.