Question

Alan is trying to throw a ball across a 50m wide river 100m below him. Alan knows physics so he throws the ball at a 45 degree upward angle at 30m/s for maximum distance. Will the ball cross the river?

Answer 1

yes

Step-by-step explanation:

u = 30 m/s

θ = 45°

h = - 100 m (below)

d = 50 m

g = - 9.8 m/s^2

Use second equation of motion in vertical direction

`h=u_(y)t +(1)/(2)a_(y)t^(2)`

`-100=30* Sin45* t -0.5* 9.8t^(2)`

`-100 = 21.21 * t -4.9 * t^(2)`

`t=\frac{21.21\pm \sqrt{21.21^(2)+4*4.9 * 100}}{9.8}`

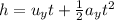
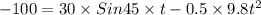
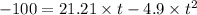
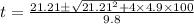
By solving we get

t = 7.17 s

The horizontal distance traveled in this time

= u Cos45 x t = 30 x 0.707 x 7.17 = 152.1 m

This distance is more than the width of the river, So the ball crosses the river.