Question

The vector y = ai + bj is perpendicular to the line ax + by = c. Use this fact to find an equation of the line through P perpendicular to v. Then draw a sketch of the line including v as a vector starting at the origin. P(-1,-9)v = -4i + j The equation of the line is _____.

Answer 1

`-4x+y=-5`

Explanation:

It is given that he vector y = ai + bj is perpendicular to the line ax + by = c.

Given given point is P(-1,-9) and given vector is v = -4i + j.

Here, a = -4 and b= 1.

We need to find the equation of the line through P perpendicular to v.

Using the above fact, the vector v = -4i + j is perpendicular to the line

`(-4)x+(1)y=c`

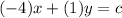
`-4x+y=c` ... (1)

The line passes through the point (-1,-9).

Substitute x=-1 and y=-9 in the above equation to find the value of c.

`-4(-1)+(-9)=c`

`4-9=c`

`-5=c`

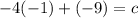
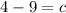
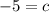
The value of c is -5.

Substitute c=-5 in equation (1).

`-4x+y=-5`

Therefore the equation of line which passes through P and perpendicular to v is -4x+y=-5.