Question

If we assume the population of Grand Rapids is growing at a rate of approximately 4% per decade, we can model the population function with the formula

P( t ) = 181843 ( 1.04 )^(t / 10).
Use this formula to compute the average rate of change of the population on the intervals [ 5 , 10 ] , [ 5 , 9 ] , [ 5 , 8 ] , [ 5 , 7 ] , and [ 5 , 6 ] .

Answer 1

The average rate of change of the population on the intervals [ 5 , 10 ] , [ 5 , 9 ] , [ 5 , 8 ] , [ 5 , 7 ] , and [ 5 , 6 ] are 734.504, 733.06, 731.62, 730.185 and 728.75 respectively.

Explanation:

The given function is

`P(t)=181843(1.04)^{((t)/(10))}`

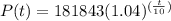
where, P(t) is population after t years.

At t=5,

`P(5)=181843(1.04)^{((5)/(10))}=185444.20`

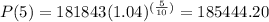
At t=6,

`P(6)=181843(1.04)^{((6)/(10))}=186172.95`

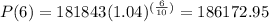
At t=7,

`P(7)=181843(1.04)^{((7)/(10))}=186904.57`

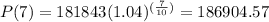
At t=8,

`P(8)=181843(1.04)^{((8)/(10))}=187639.06`

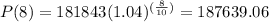
At t=9,

`P(9)=181843(1.04)^{((9)/(10))}=188376.44`

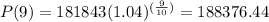
At t=10,

`P(10)=181843(1.04)^{((10)/(10))}=189116.72`

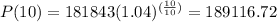
The rate of change of P(t) on the interval
`[x_1,x_2]` is

`m=(P(x_2)-P(x_1))/(x_2-x_1)`

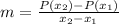
Using the above formula, the average rate of change of the population on the intervals [ 5 , 10 ] is

`m=(P(10)-P(5))/(10-5)=(189116.72-185444.20)/(5)=734.504`

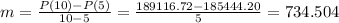
The average rate of change of the population on the intervals [ 5 , 9 ] is

`m=(P(9)-P(5))/(9-5)=(188376.44-185444.20)/(4)=733.06`

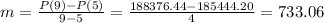
The average rate of change of the population on the intervals [ 5 , 8 ] is

`m=(P(8)-P(5))/(8-5)=(187639.06-185444.20)/(3)=731.62`

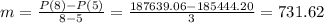
The average rate of change of the population on the intervals [ 5 , 7 ] is

`m=(P(7)-P(5))/(7-5)=(186904.57-185444.20)/(2)=730.185`

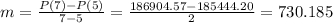
The average rate of change of the population on the intervals [ 5 , 6 ] is

`m=(P(6)-P(5))/(6-5)=(186172.95-185444.20)/(1)=728.75`

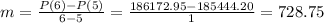
Therefore the average rate of change of the population on the intervals [ 5 , 10 ] , [ 5 , 9 ] , [ 5 , 8 ] , [ 5 , 7 ] , and [ 5 , 6 ] are 734.504, 733.06, 731.62, 730.185 and 728.75 respectively.