Question

An imaginary element (X) on Mars is composed of three isotopes, 10.68% of isotope X-95 with a mass of 95.0 amu, 16.90% of isotope X-96 with a mass of 96.0 amu, and 72.42% of isotope X-97 with a mass of 97.0 amu. Calculate the atomic mass (in amu) of the element. Type in your answer with 3 significant figures.

Answer 1

To calculate the atomic mass of the element with three isotopes, you multiply the percentage abundance by the mass of each isotope and then add the values.

Step-by-step explanation:

To calculate the atomic mass of the element, we need to multiply the percentage abundance of each isotope by its respective mass and then sum up the products.

For isotope X-95: (10.68/100) x 95.0 amu = 10.1376 amu

For isotope X-96: (16.90/100) x 96.0 amu = 16.224 amu

For isotope X-97: (72.42/100) x 97.0 amu = 70.2774 amu

Adding the values: 10.1376 amu + 16.224 amu + 70.2774 amu = 96.639 amu

Therefore, the atomic mass of the element is 96.639 amu (rounded to 3 significant figures).

Answer 2

Answer: The average atomic mass of element X is 96.6 amu.

Step-by-step explanation:

Average atomic mass of an element is defined as the sum of masses of each isotope each multiplied by their natural fractional abundance.

Formula used to calculate average atomic mass follows:

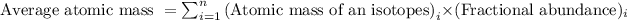`\text{Average atomic mass }=\sum_(i=1)^n\text{(Atomic mass of an isotopes)}_i* \text{(Fractional abundance})_i` .....(1)

• For isotope 1 (X-95):

Mass of isotope 1 = 95.0 amu

Percentage abundance of isotope 1 = 10.68 %

Fractional abundance of isotope 1 = 0.1068

• For isotope 2 (X-96):

Mass of isotope 2 = 96.0 amu

Percentage abundance of isotope 2 = 16.90 %

Fractional abundance of isotope 2 = 0.1690

• For isotope 3 (X-97):

Mass of isotope 3 = 97.0 amu

Percentage abundance of isotope 3 = 72.42 %

Fractional abundance of isotope 3 = 0.7242

Putting values in equation 1, we get:

`\text{Average atomic mass of X}=[(95* 0.1068)+(96* 0.1690)+(97* 0.7242)]`

`\text{Average atomic mass of X}=96.6amu`

Hence, the average atomic mass of element X is 96.6 amu.