Question

Set A contains three different positive odd integers and two different positive even integers; set B contains two different positive odd integers and three different positive even integers. If one integer from set A and one integer from set B are chosen at random, what is the probability that the product of the chosen integers is even?

Answer 1

`(2)/(5)*(3)/(5) +(2)/(5)*(2)/(5)+(3)/(5) *(3)/(5)  = (19)/(25)`

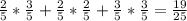
Explanation:

We must remember that in order to get one even number we need to multiply one even number times one odd number or two even numbers. So, the first term tells the probability of having an even number from A and an even number from B, the next would be even from A and odd from B and the last one tells the likelihood of having odd from A and even from B