Question

An air hockey game has a puck of mass 30 grams and a diameter of 100 mm. The air film under the puck is 0.1 mm thick. Calculate the time required after impact for a puck to lose 10% of its initial speed. Assume air is at 15o C and has a dynamic viscosity of 1.75´10-5 N×s/m2 .

Answer 1

time required after impact for a puck is 2.18 seconds

Step-by-step explanation:

given data

mass = 30 g = 0.03 kg

diameter = 100 mm = 0.1 m

thick = 0.1 mm = 1 ×
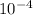`10^(-4)` m

dynamic viscosity = 1.75 ×
`10^(-5)` Ns/m²

air temperature = 15°C

to find out

time required after impact for a puck to lose 10%

solution

we know velocity varies here 0 to v

we consider here initial velocity = v

so final velocity = 0.9v

so change in velocity is du = v

and clearance dy = h

and shear stress acting on surface is here express as

= µ
`(du)/(dy)`

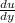
so

= µ
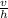`(v)/(h)` ............1

put here value

= 1.75×
`10^(-5)` ×
`(v)/(10^(-4))`

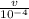
= 0.175 v

and

area between air and puck is given by

Area =
`(\pi )/(4) d^(2)`

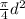
area =
`(\pi )/(4) 0.1^(2)`

area = 7.85 ×
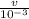`(v)/(10^(-3))` m²

so

force on puck is express as

Force = × area

force = 0.175 v × 7.85 ×
`10^(-3)`

force = 1.374 ×
`10^(-3)` v

and now apply newton second law

force = mass × acceleration

- force =
`mass (dv)/(dt)`

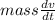
- 1.374 ×
`10^(-3)` v =
`0.03 (0.9v - v )/(t)`

t =
`(0.1 v * 0.03)/(1.37*10^(-3) v)`

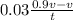
time = 2.18

so time required after impact for a puck is 2.18 seconds