Question

Accelerating charges radiate electromagnetic waves. Calculate the wavelength of radiation produced by a proton of mass mp moving in a circular path perpendicular to a magnetic field of magnitude B. (Use any variable or symbol stated above along with the following as necessary: q and c.)

Answer 1

Step-by-step explanation:

Let
`m_p` is the mass of proton. It is moving in a circular path perpendicular to a magnetic field of magnitude B.

The magnetic force is balanced by the centripetal force acting on the proton as :

`(mv^2)/(r)=qvB`

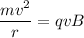
r is the radius of path,

`r=(mv)/(qB)`

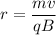
Time period is given by :

`T=(2\pi r)/(v)`

`T=(2\pi m_p)/(qB)`

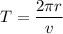
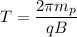
Frequency of proton is given by :

`f=(1)/(T)=(qB)/(2\pi m_p)`

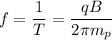
The wavelength of radiation is given by :

`\lambda=(c)/(f)`

`\lambda=(2\pi m_pc)/(qB)`

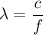
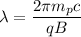
So, the wavelength of radiation produced by a proton is
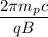`(2\pi m_pc)/(qB)`. Hence, this is the required solution.