Question

A cylinder has a volume of 198 cm3, and its base has an area of 22 cm2. What is the height of the cylinder? V= πr²h

Answer 1

The height of cylinder is 9 cm

Explanation:

Volume of cylinder = 198 cm³

Base Area = 22 cm²

We need to find height of the cylinder

The formula used is:
`Volume\:of\:cylinder=\pi r^2h`

Where
`Base\: Area = \pi r^2`

First we need to find radius r

`Base\: Area = \pi r^2\\22=3.14\: r^2\\r^2=(22)/(3.14)\\r^2=7\\Taking\: square\: root\: on\: both\: sides\\√(r^2)=√(7)\\r=2.656`

So, we get radius r = 2.656

Putting values of volume and radius are to find height

`Volume\:of\:cylinder=\pi r^2h\\198=3.14(2.656)^2h\\h=(198)/(22)\\h=9`

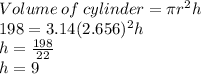
So, the height of cylinder is 9 cm