Question

What is the slope of a line that is perpendicular to the line in the graph?

Answer 1

The slope of the required line is 1

Explanation:

The Slope of a Line

Suppose we know the line passes through points A(x1,y1) and B(x2,y2). The slope can be calculated with the equation:

`\displaystyle m=(y_2-y_1)/(x_2-x_1)`

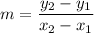
The graph shows a line whose slope can be calculated by selecting two points it goes through. Let's pick points (0,0) and (2,-2). Thus the slope is:

`\displaystyle m=(-2-0)/(2-0)=-1`

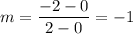
The slope (m') of a line that is perpendicular to the line in the graph can be found by using the equation:

m*m'=-1

Solving for m':

`m'=-(1)/(m)=-(1)/(-1)=1`

Thus the slope of the required line is 1