Question

I need help if you can that would be great thank you

Answer 1

Simplifying the expression
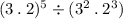`(3\:.\:2)^5/ (3^2\:.\:2^3)` so, there is only one power of each base we get
`\mathbf{3^3\:.\:2^2}`

Option D is correct answer.

Explanation:

We need to simply the expression
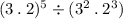`(3\:.\:2)^5/ (3^2\:.\:2^3)` so, there is only one power of each base.

Solving:

`(3\:.\:2)^5/ (3^2\:.\:2^3)`

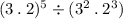
We can write it as:

`((3\:.\:2)^5)/(3^2.2^3)`

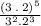
Now using exponent rule:
`(a\:.\:b)^m=a^m\:.\:b^n`

`(3^5\:.\:2^5)/(3^2.2^3)`

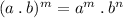
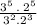
Now using the exponent rule:
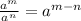`(a^m)/(a^n)=a^(m-n)` the bases should be same

`3^(5-2)\:.\:2^(5-3)\\=3^3\:.\:2^2`

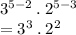
So, simplifying the expression
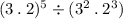`(3\:.\:2)^5/ (3^2\:.\:2^3)` so, there is only one power of each base we get
`\mathbf{3^3\:.\:2^2}`

Option D is correct answer.