Answer:
The plane will be 4795.23 meters from where he threw the package.
Step-by-step explanation:
Data:
v = 167 m/s
h = 4040 m
g = 9.8m/s²
package:
after it is dropped there is no horizontal force, so...

t =
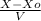 I
I
in the vertical we have only
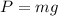
 but Vo = 0 because the package is dropped
but Vo = 0 because the package is dropped
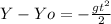 II
II
replacing I in II
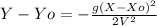

X - Xo = 4795.23
The distance from where the plane drops the package and where it hits the ground is the same as the plane flies horizontally, as there is no acceleration at x.