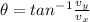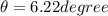Question

A plane flying from Townsville, Australia, has an air speed of 264 m/s in a direction 5.0° south of west. It is in the jet stream, which is blowing at 37 m/s in a direction 15° south of east. In this problem you are going to be asked about the velocity of the airplane relative to the Earth.

Answer 1

Speed of plane is 300.5 m/s at angle of 6.22 degree South of West

Step-by-step explanation:

Air speed of the plane is given as

v = 264 m/s in direction 5 degree South of West

So we have

`v_1 = 264 cos5 \hat i + 264 sin5 \hat j`

`v_1 = 263 \hat i + 23 \hat j`

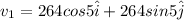
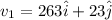
Also we have speed of air is given as

v = 37 m/s at 15 degree South of West

so it is

`v_2 = 37 cos15\hat i + 37 sin15 \hat j`

`v_2 = 35.74 \hat i + 9.58 \hat j`

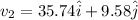
So the net speed of plane with respect to ground is given as

`v_p = v_1 + v_2`

`v_p = (263 \hat i + 23 \hat j) + (35.74 \hat i + 9.58 \hat j)`

`v_p = 298.74 \hat i + 32.58\hat j`

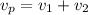
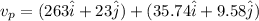
so it is

`v_p = √(298.74^2 + 32.58^2)`

`v_p = 300.5 m/s`

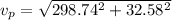
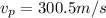
direction is given as

`\theta =tan^(-1) (v_y)/(v_x)`

`\theta = tan^(-1) (32.58)/(298.74)`

`\theta = 6.22 degree`