Question

If BA [a= (-2,-3) b= (1,2)] is extended all the way through A creating a BF and A becomes the midpoint of BF, then what are the coordinates

Answer 1

(-5,-8)

Explanation:

If M(x,y) is the midpoint of the segment CD, where
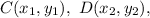`C(x_1,y_1),\ D(x_2,y_2),` then

`x=(x_1+x_2)/(2)\\ \\y=(y_1+y_2)/(2)`

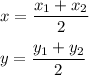
You are given two points A(-2,-3) and B(1,2), let point F be the point with coordinates (x,y). Yuo know, that point A is the midpoint of BF, then

`x_A=(x_B+x_F)/(2)\\ \\y_A=(y_B+y_F)/(2)`

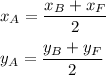
Substitute known coordinates:

`-2=(1+x)/(2)\Rightarrow 1+x=-4,\ \ x=-5\\ \\-3=(2+y)/(2)\Rightarrow 2+y=-6,\ \ y=-8`

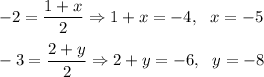
So, point F has coordinates (-5,-8)