Answer:
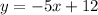 or
or
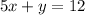
Explanation:
The given equation of a line:
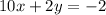
Rewrite equation of line in slope intercept form (y=mx+c) by subtracting 10 on both sides and then divide both sides by 2, we get
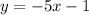
Slope of line : m= -5 [Coefficient of x]
Since , the slope of two parallel lines are equal.
Then, slope of line parallel to given line= -5
Equation of a line passing through point (a,b) and has slope p is given by :-
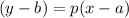
Similarly, Equation of a line passing through point (0,12) and has slope -5 is given by :-
