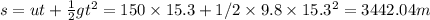Question

A rocket moves upward, starting from rest with an acceleration of +30.0 m/s2 for 5.00 s. It runs out of fuel at the end of this 5.00 s and continues to move upward. How high does it rise? m

Answer 1

The distance covered by the rocket after fuel ran out is
`3442.04 m`

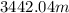
Step-by-step explanation:

Given that the rocket moves with an acceleration
`a=30m/s^2`

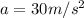
time
`t=5 s`

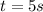
Since the rocket starts from rest initial velocity
`u=0 s`

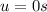
The distance it travelled within this time is given by
`s=ut+ (1)/(2) at^2`
`=0 * 5+ (1)/(2) (30*25)=375 m`

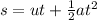
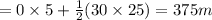
Velocity at this point is given by
`v=u+at`

`v=0+30*5=150m/s`

Given that at this height it runs out of fuel but travels further. Here final velocity
`v=0`(maximum height), initial velocity
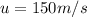`u=150 m/s` and time to zero velocity
`t=(v)/(g) = (150)/(9.8) =15.3 s.`

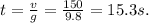
Thus it travels
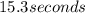`15.3 seconds` more after fuel running out. The distance covered during this period is given

`s= ut+(1)/(2) gt^2=150 * 15.3+1/2 *9.8 * 15.3^2=3442.04 m`