Answer: (a) $197,500
(b) $ 189,500
Step-by-step explanation:
Given : The marginal cost function :
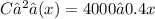
To find the cost function, we need to integrate the above function with respect to x.
Now, the additional cost incurred in dollars when production is increased from 100 units to 150 units will be:-
![\int^(150)_(100)\ C'(x)\ dx\\\\=\int^(150)_(100) (4000-0.4x)\ dx\\\\=[4000x-(0.4x^2)/(2)]^(150)_(100)\\\\=[4000(150)-(0.4(150)^2)/(2)-4000(100)+(0.4(100)^2)/(2)]\\\\=[600000-4500-400000+2000]\\\\=197500](https://img.qammunity.org/2020/formulas/business/college/l5se1324zry6j3bookvtqasfxu0j0yhb3y.png)
Hence, the additional cost incurred in dollars when production is increased from 100 units to 150 units= $197,500
Similarly, the additional cost incurred in dollars when production is increased from 500 units to 550 units :-
![\int^(550)_(500)\ C'(x)\ dx\\\\=\int^(550)_(500) (4000-0.4x)\ dx\\\\=[4000x-(0.4x^2)/(2)]^(550)_(500)\\\\=[4000(550)-(0.4(550)^2)/(2)-4000(500)+(0.4(500)^2)/(2)]\\\\=[2200000-60500-2000000+50000]\\\\=189,500](https://img.qammunity.org/2020/formulas/business/college/7n1of0z15t2smjc7ln00gv5vbhjmrpus5k.png)
Hence, the additional cost incurred in dollars when production is increased from 500 units to 550 units = $ 189,500