Question

In Mary's first math test she scored 87%. The mean and standard deviation for the class were 71% and 18% respectively. In her second math test, Mary scored 66%. The mean and standard deviation for the class were 53% and 14% respectively. In which test did Mary do better relative to the rest of the class? Explain your reasoning. (Hint: find the z-scores corresponding to her two test scores.)

Answer 1

Explanation:

Since your population are the students in math class, you can use the z-score formula
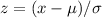`z=(x-\mu)/\sigma` in order to comparing the two math test scores. Where
`\mu` is the mean for the class,
`\sigma` is the standars deviation and x is Mary score.

For the first test
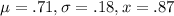`\mu=.71 , \sigma=.18,x=.87` , so ,
`z_(1) = (.87-.71)/(.18)=.88`.

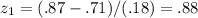For the second test
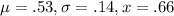`\mu=.53 , \sigma=.14,x=.66` , so ,
`z_(1) = (.66-.53)/(.14)=.93`

Mary do better in the second test, relative to the rest of the class (because
`.88 \leq .93`, it means the second score is nearer to the mean score of the class than the first one )