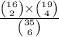Question

A class has 35 students, of which 16 are male and 19 are female. If 6 of the students are selected at random to form a committee, what is the probability that exactly 2 male students are selected?

Answer 1

The probability of choosing exactly 2 male and 4 female students =
`\frac{\binom{16}{2}* \binom{19}{4}}{\binom{35}{6}}`

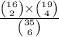
Explanation:

We are given that a class has 35 students

Number of male=16

Number of female=19

We have to choose 6 students for committee

We have to find the probability that exactly 2 male students are selected

Probability=P(E)=
`(number\;of\;favorable\;cases)/(total\;number\;of\;cases)`

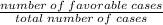
If we have to choose total 6 student in which 2 male and 4 female

Combination formula:

`nC_r=(n!)/(r!(n-r)!)`

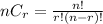
Using the formula

The probability of choosing exactly 2 male and 4 female students =
`\frac{\binom{16}{2}* \binom{19}{4}}{\binom{35}{6}}`