Question

I NEED ANSWER ASAP

A block of ice has the density of 0.97 g/cm3 . How much of it will sink below the surface of the water?

About 97 percent of the ice will sink below water.


All—100 percent—of the ice will sink below water.


About 3 percent of the ice will sink below water.


None—0 percent—of the ice will sink below the water.

Answer 1

97% will sink below the water

Step-by-step explanation:

Waters density is 1 g/cm3

If an object's density is greater than 1g/cm3, it will sink. If it's less then it would float. 1-0.97 = 0.03. Only 3% of the ice would show while 97 will be under.

Answer 2

About 97 percent of the ice will sink below water.

Step-by-step explanation:

Let the volume that is submerged into the water is given as V1

so at equilibrium condition we can say that buoyancy force on it is balanced by weight of the block

so we have

`\pho_(water) V_1 g = \rho_(ice) V g`

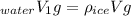
now we have

`(V_1)/(V) = (\rho_(ice))/(\rho_(water))`

`(V_1)/(V) = (0.97)/(1)`

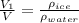
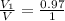
so the percentage of block that is submerged into the water is given as

volume percent submerged = 97 %