Answer:
A 95% confidence interval for the mean number of months elapsed since the last visit to a dentist, is
![[5.79063, 28.20937]](https://img.qammunity.org/2020/formulas/mathematics/college/3yjmj4ninbq95ily3wepxwjf9rn3x46n9j.png)
Explanation:
We will build a 95% confidence interval for the mean number of months elapsed since the last visit to a dentist. A (1 -
 ) x100% confidence interval for the mean number of months elapsed since the last visit to a dentist with unknown variance and is given by:
) x100% confidence interval for the mean number of months elapsed since the last visit to a dentist with unknown variance and is given by:
![[\bar x -T_{(n-1,(\alpha)/(2))} (S)/(√(n)), \bar x +T_{(n-1,(\alpha)/(2))} (S)/(√(n))]](https://img.qammunity.org/2020/formulas/mathematics/college/5yo5b8pey2r7pj0v58cadnvd1xda01aplk.png)

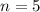
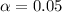
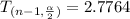
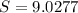
![[17 -2.7764 (9.0277)/(√(5)), 17 +2.77644 (9.0277)/(√(5))]](https://img.qammunity.org/2020/formulas/mathematics/college/a23pjex5b9u960eqt2n35yt0scw40lqlxq.png)
A 95% confidence interval for the mean number of months elapsed since the last visit to a dentist, is
![[5.79063, 28.20937]](https://img.qammunity.org/2020/formulas/mathematics/college/3yjmj4ninbq95ily3wepxwjf9rn3x46n9j.png)